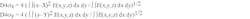BEAM CHARACTERIZATION: Camera-based sensors characterize laser beams
CARLOS ROUNDY, GREG SLOBODZIAN, and LARRY GREEN
The beam profile is of critical importance for many laser applications. For some applications, beam-profile measurement is necessary only during the design or fabrication phase of the laser, but for others, the beam profile must be continuously monitored during laser operation. For example, some industrial laser applications require periodic beam-profile monitoring to eliminate scrap that can be produced when the laser degrades. In medical applications, the practitioner cannot tune the laser, so the manufacturer measures the beam profile during design to ensure it provides reliable performance at all times. However, in some medical uses of lasers, such as laser-assisted in situ keratomileusis (LASIK), periodic checking of the beam profile can considerably enhance the reliability of the operation.
The beam profile is significant because it affects the energy density, the concentration, and the collimation of the light. Also, the propagation of the beam through space is significantly affected by the beam profile. As a variety of profiles exist, it is essential to measure the profile with accuracy in any application in which the energy distribution affects the performance of the laser for its intended purpose.
Several nonelectronic measurement methods can be used to view beam profiles (see Fig. 1). In each of these cases, however, a crude qualitative idea of the beam intensity pattern is obtained but quantitative information is lacking.
Who cares about mode?
A 100 W laser works better than a 100 W light bulb for drilling holes because its light energy is concentrated. But how much better depends on the energy distribution of the modes in the laser beam. While some laser applications require uniform flattop beams, or doughnut-mode beams with a hole in the center of a ring, most applications require a Gaussian-type beam, in which the energy is concentrated in the center of the beam.
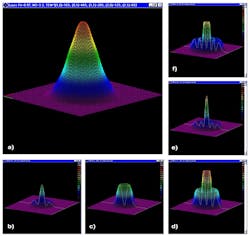
A pure Gaussian beam is –designated TEM00 and focuses to a spot smaller than any other beam. However, a pure TEM00 beam does not exist in practice. How close a beam comes to the ideal is expressed by a factor called "times-diffraction-limit" or M squared (M2), where M2 = 1 is a perfect TEM00 beam and M2 > 1 contains other laser modes that reduce its ability to focus. While a "Gaussian fit" of a laser beam is sometimes a good first approximation of an MTo understand modes, one can look at a computer-generated laser beam with a 97% Gaussian fit, but with an M2 = 3.3, much greater than 1 (see Fig. 2). This beam is composed of zero percent TEM00 mode, but instead contains 16% TEM10 mode, 44% TEM01, 20% TEM11, 12% TEM20, and 8% TEM21 mode contributions (see www.laserfocusworld.com/articles/54463).
Another dramatic example of modes and beam profiles is apparent when looking at beams from operating lasers. An Nd:YAG laser operating at 100 W produces a quasi-Gaussian beam. When the power is increased to 170 W to increase material penetration depth, the beam splits into two peaks. Instead of the power density increasing by 70% using the simple calculation (170 W – 100 W)/100 W, the power density actually decreased by 13% due to broadening of the beam profile.
With such dramatic mode changes as a function of power level, measuring the beam width and power density, and viewing the beam profile are all that is required for some rudimentary applications. However, for more exacting applications in which the difference between an M2 = 1.1 and M2 = 1.3 can result in success or failure, a more intricate profile measurement system is required.
Mode measurement
In electronic beam-profile measurement, the beam is typically attenuated using beamsplitters to direct a small portion of the beam toward the sensor, followed by neutral-density filters for further attenuation. While various types of mechanical scanning beam-profiler sensors exist—each with its own advantages for certain applications—most beam-profiling applications use cameras for the sensor. The attenuated beam is projected directly onto the two-dimensional (2-D) camera sensor such that each camera pixel measures the intensity of that part of the beam striking it. Reading these signals into a computer, which converts them into either 2-D or 3-D displays, allows the user to "see" the beam profile with great clarity and precision.
Cameras have the distinct advantage of providing an instantaneous view of the entire 2-D beam profile. Charge-coupled-device (CCD) cameras are typically used for visible and near-infrared lasers with wavelengths up to 1.1 µm. Cameras with indium gallium arsenide (InGaAs) sensors and phosphor-coated CCDs are used in the 1.5 µm telecom and laser-rangefinder wavelength range. Pyroelectric solid-state cameras are used for wavelengths from 1.1 µm to greater than 1000 µm.
Commercial suppliers of beam profilers provide a wide array of camera choices and beam attenuation choices to fit a wide variety of applications. Currently, most CCD cameras have a FireWire or a USB-2 interface, which enables them to connect easily with laptop computers for portable applications. Measuring M2 requires, in addition, an optical train to enable measurement through the beam waist.1
Software: the critical element
Computer software takes the string of pixel data from the camera sensor to create the displays and quantitative data needed to properly analyze a laser beam. Well-written software creates clear, concise displays of the beam profile, with easy-to-use options of 2-D and 3-D rendering. Just seeing the 2-D beam profile often provides all that a user needs to be able to optimize the laser.
The most important quantitative measurement of a beam profile provided by the software is the beam width. Over the years there have been various definitions of beam width, which have arisen because a laser beam does not have sharp boundaries, but instead has tailing wings that make it hard to measure. Examples that have all but disappeared include percent of peak, percent of energy, defined aperture, power in the bucket, 1/e2 width, and full-width-half-maximum (FWHM). The definition that has emerged as the most useful is the width calculated by the second-moment integral:
Here, ( x– X) and ( y– Y) are the distances to the beam centroids X and Y, defined as the center of mass of the laser beam and calculated as:
The second-moment integral equations yield the ± 2s width that contains 86% of the beam energy and is a common statistical relationship in natural phenomena. D4s is the most useful width definition because it correctly calculates the 1/e2 beam width that survives in the beam propagation equation to predict future beam properties. Also useful is a 10% to 90% knife-edge width multiplied by a constant to approximate the D4s.
Nevertheless, calculating D4s can be tricky using cameras. All cameras have a baseline offset, which is the difference between the camera output with zero input signal and the digitizer zero level. This offset must be correctly processed or significant errors can occur. For example, if the baseline offset is positive, the calculated beam width is erroneously calculated to be much too large because the algorithm incorrectly thinks that the offset is energy in the wings of the beam. If the offset is negative then the wings of the beam are "cut off" by the processor and the calculated beam width will be too small. Look for beam profilers that provide special algorithms that correctly account for the baseline and correctly process random noise to provide a precision beam-width calculation.
REFERENCE
1. C. Roundy, Industrial Laser Solutions 15(2) (February 2000).
CARLOS ROUNDY is Spiricon founder emeritus, GREG SLOBODZIAN is vice president, engineering, and LARRY GREEN is industrial-product sales manager for Ophir-Spiricon's Laser Measurement Group, 60 West 1000 North, Logan, UT 84321; e-mail: [email protected]; www.ophir-spiricon.com.