Navigating Spherical Mirrors: A Comprehensive Overview
Understanding the intricacies of spherical mirrors is crucial for comprehending how light behaves when reflected. This guide offers a detailed exploration of concave and convex mirrors, shedding light on their distinct characteristics and applications.
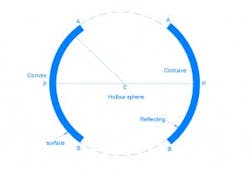
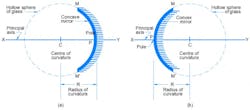
Spherical mirrors are categorized into two types: concave mirrors and convex mirrors. Concave mirrors curve inward, converging parallel rays of light, while convex mirrors curve outward, causing parallel rays to diverge.
Key Terminologies:
- Center of Curvature (C): The center of the hollow sphere, representing a pivotal point for understanding the mirror’s behavior.
- Pole (P): The central point of the spherical mirror, distinct from the center of curvature.
- Radius of Curvature: The distance between the center of curvature and the pole.
- Principal Axis: A straight line connecting the pole and the center of the mirror.
- Focal Length (f): Half the distance of the radius of curvature, determining the mirror’s focusing capability.
Sign Conventions
Sign conventions play a crucial role in deriving formulae for reflection and refraction by spherical mirrors and lenses. They dictate that distances measured in the direction of incident light are positive, while heights measured upward normal to the principal axis are positive.
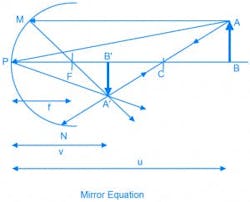
Mirror Equation
The mirror equation simplifies the formation of images by spherical mirrors. It relates the object distance (u), image distance (v), and focal length (f), providing a practical tool for understanding image formation.
Consider an object positioned at a distance ‘u’ from the pole of the mirror. Reflected rays converge to form an inverted image at a distance ‘v’ from the pole. The mirror equation, along with diagrams, illustrates this phenomenon, enhancing the understanding of theoretical concepts.
Contact Shanghai Optics today! We’d be more than happy to discuss your projects and how to best bring them to fruition.